Structure functions

Perturbative QCD (pQCD) does not predict the absolute value of the parton densities within the proton but determines how they vary from a given input. For a given initial distribution at a particular scale, Q₀²,  Altarelli-Parisi (DGLAP) evolution [4] enables the distributions at higher Q² to be determined. DGLAP evolution resums the leading log(Q²) contributions associated with a chain of gluon emissions. At large enough positron-proton centre-of-mass energies there is a second large variable 1/x and, therefore, it is also necessary to resum the log(1/x) contributions. This is achieved by using the BFKL equation [5]. In the DGLAP parton evolution scheme the parton cascade follows a strong ordering in transverse momentum p_Tn² $\gg$ p_{Tn - 1}² $\gg$ ... $\gg$ p_T1², while there is only a soft (kinematical) ordering for the fractional momentum x_n < x_{n - 1} < ... < x₁ (see figure 2.) By contrast, in the BFKL scheme the cascade follows a strong ordering in fractional momentum x_n $\ll$ x_{n - 1} $\ll$ ... $\ll$ x₁, while there is no ordering in transverse momentum.

At small x the dominant parton is the gluon and the description of the structure function is driven by the behaviour of the gluon. Because of gluon splitting, g $\rightarrow$ q$\bar{q}$, pQCD suggests the small x behaviour of the sea quark and gluon distributions are strongly correlated.

The kinematic plane covered by HERA and the fixed target measurements is shown in fig. 3. HERA has increased the reach in Q² by about 2 orders of magnitude and can also probe nearly 3 orders of magnitude further down in x. The low x region is correlated with low values of Q². The differential NC DIS cross section is related to three structure functions:

$${\frac{d^2\sigma^{e^{\pm}p}}{dxdQ^2}} {\frac{2\pi\alpha^2}{xQ^4}} \mp$$ (1)

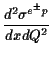where Y_$\pm$ = 1$\pm$(1 - y)². The structure function F₂ in QPM is just the sum of the quark densities multiplied by the appropriate electric charge; F₃ arises from the weak part of the cross section and is negligible for Q² < 5000 GeV², and F_L is the longitudinal structure function and only becomes important for y > 0.6. Hence by measuring the differential cross section at HERA one is effectively measuring the structure function F₂.

The F₂ measurements are shown in figs. 4 and 5 as a function of x and Q² respectively. The error bars are at the 5-10% level and the normalisation uncertainty is $\sim$2%. There is a steep rise of F₂ with decreasing x in all Q² bins, fig. 4. Scaling violations in Q² are clearly seen in fig. 5. Both H1 and ZEUS have performed next to leading order (NLO) QCD fits [6,7] based on the DGLAP evolution equations using both HERA and fixed target data. Fig. 5 shows that these QCD fits describe the F₂ data well, though it should be noted that the data can also be satisfactorily described by the BFKL prediction [8].

The scaling violations from the HERA data allow an estimate of the gluon density xg(x) at low values of x, whilst the fixed target data are used to constrain the high x region. The extracted gluon densities from the fits are shown in fig 6 for a fixed Q² = 20 GeV². The error band shows the statistical and systematic uncertainty taking into account correlations and variations in the mass of the charm quark, m_c, and the strong coupling constant, $\alpha_{s}^{}$. The results of the two HERA experiments are in good agreement and the extracted densities agree with the results of NMC [9] for large x. The resulting gluon distributions show a clear rise with decreasing x and have a 15% uncertainty at x $\sim$ 5 x 10^-4. These NLO QCD fits are also in good agreement with the global QCD analyses performed by MRS [10] and CTEQ [11], whilst the prediction from the dynamical evolution of GRV [12] is too steep for x < 10^-3.